Open Channel Flow – Manning Equation
Manning’s Equation for open channel flow is the go-to equation for open channel problems. An open channel is basically anything that flows out in the open above ground as well as pipes that are not flowing to their full capacity.
$$ Q = \frac{1.49AR^{\frac{2}{3}}\sqrt{s}}{n} $$
Q is the flow and can be in either cubic feet per second (US) or cubic meters per second (SI). When using the equation for SI units leave out the 1.49 factor!
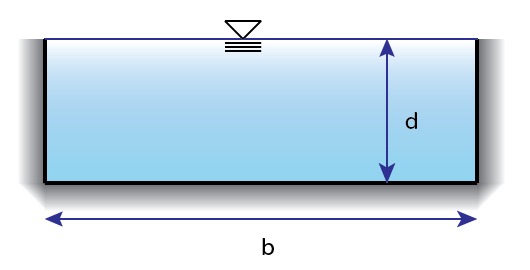
A is the Area of the channel. This can be determined with geometry for the easy rectangular and trapezoidal shapes or using table equations in combination with depth, base length, diameter etc. Find where channel geometry equations are in your reference.
R is the Hydraulic Radius. This is solved by dividing the Area (which you determined previously) by the Wetted Perimeter (P). P is the length of the wet perimeter of the channel, for a rectangular channel it is the base plus two depth lengths \(b + 2d\). The wetted perimeter is more involved for other shapes so find the table in your book for determining it for a given shape. The table in the CERM (19-3) also shows equations for R so it is handy to be aware of it.
s is the Slope of the channel. This is a decimal slope, meaning that if it is given to you in percent then convert it to decimal (2% becomes 0.02 in your equation). If it is given to you in decimal form, eg 0.025, then insert it directly in! This is confusing so remember that if a % sign or the word percent are near the slope number you should convert that to decimal form, otherwise you are ok.
n is the Manning’s Roughness Coefficient and requires a table lookup. Make sure you know where the table is in your reference! Commen values for n are 0.011-0.013.
Open channel problems often give you Q and want you to solve backward for the desired depth of a rectangular channel or diameter of a circular channel. This can be difficult because you must represent both A and R in variable terms, for example \(bd*(\frac{db}{b+2d})^{\frac{2}{3}}\). If optimum or most efficient channel is mentioned in the problem than you have been given a hint! Optimum rectangular channels have a width that is exactly twice the depth (closest in shape to a circle). This helps, and is often necessary, because you can substitute b for d, or vice versa, in your equation and eliminate a variable. These can be hard to solve and I recommend getting them to a simplified state and plugging in the multiple choice values to find the nearest solution. A quick form of trial and error.
Other Open Channel Equations
There are several other open channel related equations in all of my references, such as equations for normal depth, super/sub critical flow, critical depth, hydraulic jump and more. I don’t think I will go into those here until I figure out if they are included in the breadth portion of the exam.
Posted in Water and Environmental Breadth and tagged flow, manning equation, morning breadth, open channel flow, pe exam, water. Bookmark the permalink. Leave a Comment.


Leave a Comment
Comments (0)