Soil Mechanics – Consolidation

Source: http://www-odp.tamu.edu/publications/167_SR/chap_31/images/44_f01.gif

Consolidation is a form of settlement where the air and liquids in a soil (the voids) are removed due to pressures acting on the soil. Consolidation can occur over a long period of time and can destroy structures if not address soon enough.

The distance of consolidation is determined using this equation:

$$ s = H \varepsilon_v = \frac{H \Delta e}{1  + e_0} $$

Where:

s = settlement

H = layer thickness

\(e_0\) = initial void ratio

\(Delta e\) = change of the void ratio due to the increase in vertical stress at the midpoint of the layer

All of these are pretty straightforward. Your settlement units will be the same as your layer thickness units. Initial void ratio and change in void ratio are in decimal percent.

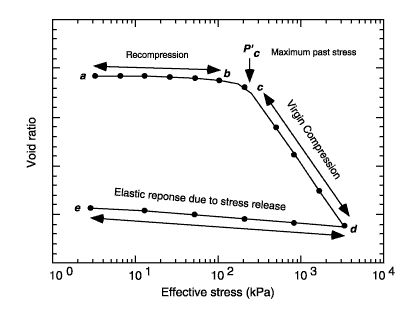
The change in the void ratio is dependent on the consolidation curve, which is pictured above (you may remember from undergrad), and the following equation:

$$ \Delta e = e_1 – e_2 = C_? \log_{10} \left( \frac{p_2′}{p_1′} ) \right) $$

The pressures (\(p\)) are the effective vertical stress at the same point, usually before, and after, a load is placed on it or before and after a change in the water table elevation (this changes effective stress remember?).

\(C_?\) should either be \(C_r\). \(C_c\) or \(C_r\) is usually given in the problem prompt so you don’t have to look to the graph to get them and you will know which what to use if there is only one. If they are both given you need to determine which one to use.

First, remember that the preconsolidation pressure (\(P_c’\)) is the dividing point between the two and corresponds to the maximum pressure that the soil has ever been exposed to. Look for that wording in a problem prompt. If the pressures at your point are HIGHER than the preconsolidation value, use \(C_c\) (this is the virgin compression line on the graph above, the soil has never been exposed to this level of pressure before). If the pressures are lower than the preconsolidation point use \(C_r\) (this is the normal or recompression line on the graph, the soils has seen this type of pressure before).

An example with numbers might look like this:

$$ \Delta e = 0.30 \log \frac{2500}{1500} = 0.067 $$

Remember, e is the change in the void ratio and is unitless.

Use delta e in the consolidation equation above and you are good to go!

The rate of consolidation can also be calculated but it is not likely to appear on the breadth portion of the exam.